Forces that move water

To observe the effects of moving a cube of water on the surrounding water, imagine a grid of uniformly spaced lines imbedded in the water (essentially a three dimensional graph paper). Move the cube to the right and the water around the cube moves with it. The force exerted by the cube on the water around it can be divided into a push, a pull, and four drags (in this two dimensional view you only see two of the four drags).
Pushing: Liquid water is almost incompressible because the molecules are so close together that there is very little "extra" space for them to move into (which is typical of all liquids). One lb/sq in will compress water about 0.0003 percent. Thus the water ahead of the cube will move almost exactly as the cube moves. If the cube of water is moved quickly a sound vibration will be generated. The sound will move at a velocity of about 1 mile / second, about 5 times the speed of sound in air. The higher speed of sound in water is another consequence of the small separation between individual water molecules.
Pulling: Water molecules are attracted to each other, so as the cube moves the water behind it will be dragged along. However, if the pulling force is too strong (too rapid), and the pressure behind the cube falls below 0.4 lbs / sq in, the water molecules will separate to form water vapor. (As the temperature increases, the pressure at which water become a gas increases; at 212 F, the pressure is 15 lb / sq in, atmospheric, the water has boiled)

It is seems unlikely that this would happen as a result of the forces that move currents. It frequently happens along the propeller blades of boats, particularly propellers that revolve rapidly. The formation of bubbles of water vapor in this situation is called cavitation. It is usually undesirable because it lowers the efficiency of propulsion and can erode metal from the propeller blade because the local forces are so large.
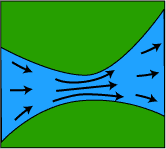
Dragging: Since water molecules attract each other, the cube will drag along the water at its sides. If the velocity is low (the definition of low follows later) the velocity of the water will linearly decrease from the cube to the edge of the container of the water, which for currents in the bay is either the bottom or the shoreline. The dragging force on the cube is proportional to the viscosity of the water and inversely proportional to the distance from the solid surface. The stream lines (if there were small particles in the water the stream lines are the paths of the particles) are parallel to the fixed surface, in the figure on the left it is the bottom of the bay.

A lamina is a thin plate, sheet, or layer. This smooth flow pattern is called laminar because the water moves as if it were made of a stack of plates slipping over each other. The alternative to laminar flow is turbulent flow. Now the water moves in a complex, changing, and basically unpredictable pattern.
The Reynolds Number

The ability to predict the velocity at which fluid flow becomes turbulent is extremely useful in the design of ships propellers, artificial hearts, and much more. Osborne Reynolds, a Professor of Engineering at Manchester, England from1868 to1905, realized that the ratio of kinetic energy to energy transferred by viscous forces was correlated with turbulent flow. When this ratio, now called the Reynolds Number, is greater than a thousand, elements of fluid "break away" from each other, i.e. the flow becomes turbulent.

The formula for the Reynolds Number is:

R = V L / v

R is the Reynolds Number
V is the velocity
L is the length
v is the kinematic viscosity = viscosity / density

If the length is 100 feet, and the velocity is 2 m / hr the Reynolds Number in water is about 30,000,000. Thus, water velocity in the bay is often 30,000 fold greater than the value associated with smooth flow. Turbulent flow is unpredictable flow, thus we can't calculate the flow, whatever the size of the computers we have.
Turbulence, unpredictability, and chaos

The idea that the motion of a fluid can not be predicted may seem a little strange. After all, don't fluids obey the laws of physics, and don't we now know how to calculate everything?. In the early 1990s there was sudden increase in understanding of turbulence, and in the more general field of chaos. The basic insight was that in systems that are chaotic, very tiny changes in the motion of a particle can result in huge changes in the future position of that particle. The tiny changes are so small that it is essentially impossible to measure or predict them, and so the future path of the particles are unpredictable. One of the several popular books written on this subject is: Chaos; making a new science by James Gleick.

While we don't know how to calculate the motion of a cube of water in turbulent flow, we do know quite a lot about the average behavior of water in turbulent flow.
Inertia: Neuton stated the most fundamental law of motion for objects: force is equal to mass times acceleration. Mass is roughly equivalent to weight and acceleration is the rate of change of velocity. Thus, if the acceleration is zero, the velocity is constant, i.e. it takes force to change velocity. Remember that velocity is both speed and direction, thus it takes force to change either speed or the direction of a moving object. Resistance to change of speed or direction is known as inertia.

This law is most easily seen in space, since the inertial force is often the only force. On earth there are many other forces that also act on an object, such as viscous drag in water, etc. However, the inertial force is always one of the forces on an object.
F = m a
Conservation: To a good approximation water is not gained or lost as it moves around in currents. Thus any set of equations that describe currents must contain express restraint. This law can most directly be seen in a channel or strait, where the water is constrained.

Another way of stating this law for a channel is that the average velocity times the cross sectional area must be a constant throughout the channel. To make it simple, suppose the depth of water is constant throughout the channel. Then the average water velocity will be greater in a narrow part of the channel (where the cross section is small) than it is in a wide part of the channel (where the cross section is large). As another example, if water is forced to flow over a shallow bar in a channel, the water velocity must increase there so that the total amount of water flowing per unit time is constant.

Return