|
In order for drug concentration measurements to have maximal impact
on drug development and clinical practice, it is necessary to
have simple models for drug transport and elimination.
Pharmacokinetic models are built out of pools.
A pool is a compartment in which mixing or diffusion is rapid
enough so that the distribution of drug throughout that pool is
essentially uniform. Pool models are used for many purposes, e.g. radioisotope labeling.
In one pool a single number represents concentration at a given
time. The pool in a pharmacokinetic model may have a clear anatomical
identity, e.g. the blood. However, a pool can also represent a
diverse collection of tissues that are combined into one model
pool with an average drug concentration. This is done when the
accuracy of the data and the needs of the analysis don't require
a more detailed model.
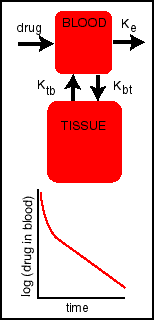
A model consists of a network of pools (the red squares on the
right) with drug transported into, out of, and between them (the
arrows). Transport and elimination is usually assumed to be first
order in concentration, i.e. a constant percent per unit time.
Thus the rate constants, Ks in the figure, have units of 1/time.
The two compartment model on the right is often used for bolus
IV administration. At t=0 the concentration in the blood is maximal.
Drug is then lost through elimination (usually by the kidneys)
and passes into the interstitial fluid in the other tissues of
the body. As the concentration in the tissue pool increases, more
drug is transported back into the blood. The return of drug to
the blood causes the rate of decrease in the blood to slow, and
thus the slope of the curve is less. Thus we see a biphasic curve.
The equation for drug concentration is the sum of two declining
exponential terms. This solution can be obtained by simple algebra, and advanced methods can solve systems of arbitrary complexity. Since solutions of
many pool models are sums of exponential terms, semi-log plots
are usually used. Then a single exponential term is a straight
line, and straight segments mean that one exponential term dominates
in that time interval. Thus, if the concentration versus time
curve were a straight line in this semi-log plot, it would indicate
only one pool, and thus suggest the drug is not transported into
tissue.
The actual shape of the curve for the IV bolus model depends on
the three rate constants (the Ks), and the ratio of volumes of
the two pools. This can be explored using a Java calculator. |